http://nextbigfuture.com/
November 18, 2015
Mark Van Raamsdonk proposes a unification of quantum mechanics and gravity. Both quantum mechanics and gravity theories have been abundantly verified through experiment, yet the realities they describe seem utterly incompatible.
Van Raamsdonk’s approach to resolving this incompatibility is strange. 'Entanglement' is the key: the phenomenon that many physicists believe to be the ultimate in quantum weirdness. Entanglement lets the measurement of one particle instantaneously determine the state of a partner particle, no matter how far away it may be — even on the other side of the Milky Way.
Entanglement might be the basis of geometry, and thus of Einstein’s geometric theory of gravity. “Space-time,” he says, “is just a geometrical picture of how stuff in the quantum system is entangled.”
This idea is a long way from being proved, and is hardly a complete theory of quantum gravity. But independent studies have reached much the same conclusion, drawing intense interest from major theorists. A small industry of physicists is now working to expand the geometry–entanglement relationship, using all the modern tools developed for quantum computing and quantum information theory.
General Relativity and Gravitation - Building up spacetime with quantum entanglement
Abstract - Building up spacetime with quantum entanglement
In this essay, we argue that the emergence of classically connected spacetimes is intimately related to the quantum entanglement of degrees of freedom in a non-perturbative description of quantum gravity. Disentangling the degrees of freedom associated with two regions of spacetime results in these regions pulling apart and pinching off from each other in a way that can be quantified by standard measures of entanglement.
Professor Mark van Raamsdonk of the University of British Columbia gave the Stanford Physics and Applied Physics Colloquium in the video belwow.
The AdS/CFT correspondence from string theory provides a quantum theory of gravity in which spacetime and gravitational physics emerge from an ordinary non-gravitational system with many degrees of freedom. In this talk, I will explain how quantum entanglement between these degrees of freedom is crucial for the emergence of a classical spacetime, and describe progress in understanding how spacetime dynamics (gravitation) arises from the physics of quantum entanglement.
Eternal black holes in anti-de Sitter by Juan Maldacena 2003
Abstract - Eternal black holes in anti-de Sitter
We propose a dual non-perturbative description for maximally extended Schwarzschild Anti-de-Sitter spacetimes. The description involves two copies of the conformal field theory associated to the AdS spacetime and an initial entangled state. In this context we also discuss a version of the information loss paradox and its resolution
Gravity without gravity
Much of this work rests on a discovery announced in 1997 by physicist Juan Maldacena, now at the Institute for Advanced Study in Princeton, New Jersey. Maldacena’s research had led him to consider the relationship between two seemingly different model universes. One is a cosmos similar to our own. Although it neither expands nor contracts, it has three dimensions, is filled with quantum particles and obeys Einstein’s equations of gravity. Known as anti-de Sitter space (AdS), it is commonly referred to as the bulk. The other model is also filled with elementary particles, but it has one dimension fewer and doesn’t recognize gravity. Commonly known as the boundary, it is a mathematically defined membrane that lies an infinite distance from any given point in the bulk, yet completely encloses it, much like the 2D surface of a balloon enclosing a 3D volume of air. The boundary particles obey the equations of a quantum system known as conformal field theory (CFT).
Maldacena discovered that the boundary and the bulk are completely equivalent. Like the 2D circuitry of a computer chip that encodes the 3D imagery of a computer game, the relatively simple, gravity-free equations that prevail on the boundary contain the same information and describe the same physics as the more complex equations that rule the bulk.
“It’s kind of a miraculous thing,” says Van Raamsdonk. Suddenly, he says, Maldacena’s duality gave physicists a way to think about quantum gravity in the bulk without thinking about gravity at all: they just had to look at the equivalent quantum state on the boundary. And in the years since, so many have rushed to explore this idea that Maldacena’s paper is now one of the most highly cited articles in physics.
Among the enthusiasts was Van Raamsdonk, who started his sabbatical by pondering one of the central unsolved questions posed by Maldacena’s discovery: exactly how does a quantum field on the boundary produce gravity in the bulk? There had already been hints3 that the answer might involve some sort of relation between geometry and entanglement. But it was unclear how significant these hints were: all the earlier work on this idea had dealt with special cases, such as a bulk universe that contained a black hole. So Van Raamsdonk decided to settle the matter, and work out whether the relationship was true in general, or was just a mathematical oddity.
He first considered an empty bulk universe, which corresponded to a single quantum field on the boundary. This field, and the quantum relationships that tied various parts of it together, contained the only entanglement in the system. But now, Van Raamsdonk wondered, what would happen to the bulk universe if that boundary entanglement were removed?
He was able to answer that question using mathematical tools4 introduced in 2006 by Shinsei Ryu, now at the University of Illinois at Urbana–Champaign, and Tadashi Takanagi, now at the Yukawa Institute for Theoretical Physics at Kyoto University in Japan. Their equations allowed him to model a slow and methodical reduction in the boundary field’s entanglement, and to watch the response in the bulk, where he saw space-time steadily elongating and pulling apart (see ‘The entanglement connection’). Ultimately, he found, reducing the entanglement to zero would break the space-time into disjointed chunks, like chewing gum stretched too far.
Quantum entanglement as geometric glue — this was the essence of Van Raamsdonk’s rejected paper and winning essay, and an idea that has increasingly resonated among physicists. No one has yet found a rigorous proof, so the idea still ranks as a conjecture. But many independent lines of reasoning support it.
In 2013, for example, Maldacena and Leonard Susskind of Stanford published a related conjecture that they dubbed ER = EPR, in honour of two landmark papers from 1935. ER, by Einstein and American-Israeli physicist Nathan Rosen, introduced what is now called a wormhole: a tunnel through space-time connecting two black holes. (No real particle could actually travel through such a wormhole, science-fiction films notwithstanding: that would require moving faster than light, which is impossible.) EPR, by Einstein, Rosen and American physicist Boris Podolsky, was the first paper to clearly articulate what is now called entanglement.
Maldacena and Susskind’s conjecture was that these two concepts are related by more than a common publication date. If any two particles are connected by entanglement, the physicists suggested, then they are effectively joined by a wormhole. And vice versa: the connection that physicists call a wormhole is equivalent to entanglement. They are different ways of describing the same underlying reality.
No one has a clear idea of what this underlying reality is. But physicists are increasingly convinced that it must exist. Maldacena, Susskind and others have been testing the ER = EPR hypothesis to see if it is mathematically consistent with everything else that is known about entanglement and wormholes — and so far, the answer is yes.
Hidden Connections
Other lines of support for the geometry–entanglement relationship have come from condensed-matter physics and quantum information theory: fields in which entanglement already plays a central part. This has allowed researchers from these disciplines to attack quantum gravity with a whole array of fresh concepts and mathematical tools
Tensor networks, for example, are a technique developed by condensed-matter physicists to track the quantum states of huge numbers of subatomic particles. Brian Swingle was using them in this way in 2007, when he was a graduate student at the Massachusetts Institute of Technology (MIT) in Cambridge, calculating how groups of electrons interact in a solid material. He found that the most useful network for this purpose started by linking adjacent pairs of electrons, which are most likely to interact with each other, then linking larger and larger groups in a pattern that resembled the hierarchy of a family tree. But then, during a course in quantum field theory, Swingle learned about Maldacena’s bulk–boundary correspondence and noticed an intriguing pattern: the mapping between the bulk and the boundary showed exactly the same tree-like network.
Swingle wondered whether this resemblance might be more than just coincidence. And in 2012, he published8 calculations showing that it was: he had independently reached much the same conclusion as Van Raamsdonk, thereby adding strong support to the geometry–entanglement idea. “You can think of space as being built from entanglement in this very precise way using the tensors,” says Swingle, who is now at Stanford and has seen tensor networks become a frequently used tool to explore the geometry–entanglement correspondence.
Another prime example of cross-fertilization is the theory of quantum error-correcting codes, which physicists invented to aid the construction of quantum computers. These machines encode information not in bits but in ‘qubits’: quantum states, such as the up or down spin of an electron, that can take on values of 1 and 0 simultaneously. In principle, when the qubits interact and become entangled in the right way, such a device could perform calculations that an ordinary computer could not finish in the lifetime of the Universe. But in practice, the process can be incredibly fragile: the slightest disturbance from the outside world will disrupt the qubits’ delicate entanglement and destroy any possibility of quantum computation.
$2.5 million funding for researching the gravity–quantum information connection
The Simons Foundation, a philanthropic organization in New York City that announced in August that it would provide US$2.5 million per year for at least 4 years to help researchers to move forward on the gravity–quantum information connection. “Information theory provides a powerful way to structure our thinking about fundamental physics,” says Patrick Hayden, the Stanford physicist who is directing the programme. He adds that the Simons sponsorship will support 16 main researchers at 14 institutions worldwide, along with students, postdocs and a series of workshops and schools. Ultimately, one major goal is to build up a comprehensive dictionary for translating geometric concepts into quantum language, and vice versa. This will hopefully help physicists to find their way to the complete theory of quantum gravity.
SOURCES - Youtube, Nature
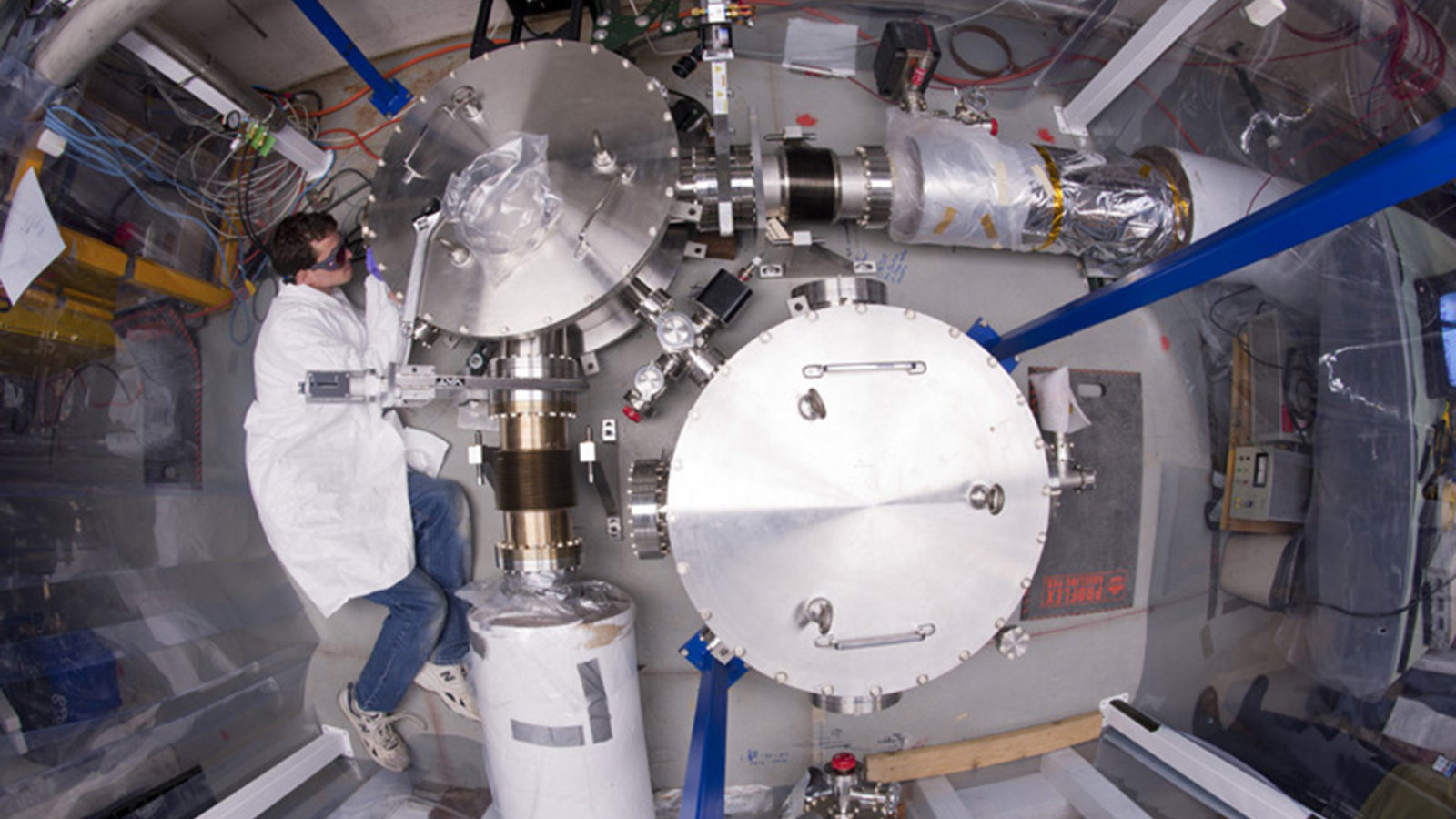
Holometer rules out first theory of space-time correlations
The extremely sensitive quantum-spacetime-measuring tool will serve as a template for continuing scientific exploration.
Our common sense and the laws of physics assume that space and
time are continuous. The Holometer, an experiment based at the US
Department of Energy’s Fermi National Accelerator Laboratory, challenges
this assumption.
We know that energy on the atomic level, for instance, is not continuous and comes in small, indivisible amounts. The Holometer was built to test if space and time behave the same way.
In a new result 1 Search for space-time correlations from the Planck scale with the Fermilab Holometer released this week after a year of data-taking, the Holometer collaboration has announced that it has ruled out one theory of a pixelated universe to a high level of statistical significance.
If space and time were not continuous, everything would be pixelated, like a digital image.
When you zoom in far enough, you see that a digital image is not smooth, but made up of individual pixels. An image can only store as much data as the number of pixels allows. If the universe were similarly segmented, then there would be a limit to the amount of information space-time could contain.
The main theory the Holometer was built to test was posited by Craig Hogan, a professor of astronomy and physics at the University of Chicago and the head of Fermilab’s Center for Particle Astrophysics. The Holometer did not detect the amount of correlated holographic noise—quantum jitter—that this particular model of space-time predicts.
But as Hogan emphasizes, it's just one theory, and with the Holometer, this team of scientists has proven that space-time can be probed at an unprecedented level.
“This is just the beginning of the story,” Hogan says. “We’ve developed a new way of studying space and time that we didn’t have before. We weren’t even sure we could attain the sensitivity we did.”
The Holometer isn’t much to look at. It’s a small array of lasers and mirrors with a trailer for a control room. But the low-tech look of the device belies the fact that it is an unprecedentedly sensitive instrument, able to measure movements that last only a millionth of a second and distances that are a billionth of a billionth of a meter—a thousand times smaller than a single proton.
The Holometer uses a pair of laser interferometers placed close to one another, each sending a 1-kilowatt beam of light through a beam splitter and down two perpendicular arms, 40 meters each. The light is then reflected back into the beam splitter where the two beams recombine.
If no motion has occurred, then the recombined beam will be the same as the original beam. But if fluctuations in brightness are observed, researchers will then analyze these fluctuations to see if the splitter is moving in a certain way, being carried along on a jitter of space itself.
According to Fermilab’s Aaron Chou, project manager of the Holometer experiment, the collaboration looked to the work done to design other, similar instruments, such as the one used in the Laser Interferometer Gravitational-Wave Observatory experiment. Chou says that once the Holometer team realized that this technology could be used to study the quantum fluctuation they were after, the work of other collaborations using laser interferometers (including LIGO) was invaluable.
“No one has ever applied this technology in this way before,” Chou says. “A small team, mostly students, built an instrument nearly as sensitive as LIGO’s to look for something completely different.”
The challenge for researchers using the Holometer is to eliminate all other sources of movement until they are left with a fluctuation they cannot explain. According to Fermilab’s Chris Stoughton, a scientist on the Holometer experiment, the process of taking data was one of constantly adjusting the machine to remove more noise.
“You would run the machine for a while, take data, and then try to get rid of all the fluctuation you could see before running it again,” he says. “The origin of the phenomenon we’re looking for is a billion billion times smaller than a proton, and the Holometer is extremely sensitive, so it picks up a lot of outside sources, such as wind and traffic.”
If the Holometer were to see holographic noise that researchers could not eliminate, it might be detecting noise that is intrinsic to space-time, which may mean that information in our universe could actually be encoded in tiny packets in two dimensions.
The fact that the Holometer ruled out his theory to a high level of significance proves that it can probe time and space at previously unimagined scales, Hogan says. It also proves that if this quantum jitter exists, it is either much smaller than the Holometer can detect, or is moving in directions the current instrument is not configured to observe.
So what’s next? Hogan says the Holometer team will continue to take and analyze data, and will publish more general and more sensitive studies of holographic noise. The collaboration already released a result related to the study of gravitational waves.
And Hogan is already putting forth a new model of holographic structure that would require similar instruments of the same sensitivity, but different configurations sensitive to the rotation of space. The Holometer, he says, will serve as a template for an entirely new field of experimental science.
“It’s new technology, and the Holometer is just the first example of a new way of studying exotic correlations,” Hogan says. “It is just the first glimpse through a newly invented microscope.”
The Holometer experiment is supported by funding from the DOE Office of Science. The Holometer collaboration includes scientists from Fermilab, the University of Chicago, the Massachusetts Institute of Technology and the University of Michigan.
We know that energy on the atomic level, for instance, is not continuous and comes in small, indivisible amounts. The Holometer was built to test if space and time behave the same way.
In a new result 1 Search for space-time correlations from the Planck scale with the Fermilab Holometer released this week after a year of data-taking, the Holometer collaboration has announced that it has ruled out one theory of a pixelated universe to a high level of statistical significance.
If space and time were not continuous, everything would be pixelated, like a digital image.
When you zoom in far enough, you see that a digital image is not smooth, but made up of individual pixels. An image can only store as much data as the number of pixels allows. If the universe were similarly segmented, then there would be a limit to the amount of information space-time could contain.
The main theory the Holometer was built to test was posited by Craig Hogan, a professor of astronomy and physics at the University of Chicago and the head of Fermilab’s Center for Particle Astrophysics. The Holometer did not detect the amount of correlated holographic noise—quantum jitter—that this particular model of space-time predicts.
But as Hogan emphasizes, it's just one theory, and with the Holometer, this team of scientists has proven that space-time can be probed at an unprecedented level.
“This is just the beginning of the story,” Hogan says. “We’ve developed a new way of studying space and time that we didn’t have before. We weren’t even sure we could attain the sensitivity we did.”
The Holometer isn’t much to look at. It’s a small array of lasers and mirrors with a trailer for a control room. But the low-tech look of the device belies the fact that it is an unprecedentedly sensitive instrument, able to measure movements that last only a millionth of a second and distances that are a billionth of a billionth of a meter—a thousand times smaller than a single proton.
The Holometer uses a pair of laser interferometers placed close to one another, each sending a 1-kilowatt beam of light through a beam splitter and down two perpendicular arms, 40 meters each. The light is then reflected back into the beam splitter where the two beams recombine.
If no motion has occurred, then the recombined beam will be the same as the original beam. But if fluctuations in brightness are observed, researchers will then analyze these fluctuations to see if the splitter is moving in a certain way, being carried along on a jitter of space itself.
According to Fermilab’s Aaron Chou, project manager of the Holometer experiment, the collaboration looked to the work done to design other, similar instruments, such as the one used in the Laser Interferometer Gravitational-Wave Observatory experiment. Chou says that once the Holometer team realized that this technology could be used to study the quantum fluctuation they were after, the work of other collaborations using laser interferometers (including LIGO) was invaluable.
“No one has ever applied this technology in this way before,” Chou says. “A small team, mostly students, built an instrument nearly as sensitive as LIGO’s to look for something completely different.”
The challenge for researchers using the Holometer is to eliminate all other sources of movement until they are left with a fluctuation they cannot explain. According to Fermilab’s Chris Stoughton, a scientist on the Holometer experiment, the process of taking data was one of constantly adjusting the machine to remove more noise.
“You would run the machine for a while, take data, and then try to get rid of all the fluctuation you could see before running it again,” he says. “The origin of the phenomenon we’re looking for is a billion billion times smaller than a proton, and the Holometer is extremely sensitive, so it picks up a lot of outside sources, such as wind and traffic.”
If the Holometer were to see holographic noise that researchers could not eliminate, it might be detecting noise that is intrinsic to space-time, which may mean that information in our universe could actually be encoded in tiny packets in two dimensions.
The fact that the Holometer ruled out his theory to a high level of significance proves that it can probe time and space at previously unimagined scales, Hogan says. It also proves that if this quantum jitter exists, it is either much smaller than the Holometer can detect, or is moving in directions the current instrument is not configured to observe.
So what’s next? Hogan says the Holometer team will continue to take and analyze data, and will publish more general and more sensitive studies of holographic noise. The collaboration already released a result related to the study of gravitational waves.
And Hogan is already putting forth a new model of holographic structure that would require similar instruments of the same sensitivity, but different configurations sensitive to the rotation of space. The Holometer, he says, will serve as a template for an entirely new field of experimental science.
“It’s new technology, and the Holometer is just the first example of a new way of studying exotic correlations,” Hogan says. “It is just the first glimpse through a newly invented microscope.”
The Holometer experiment is supported by funding from the DOE Office of Science. The Holometer collaboration includes scientists from Fermilab, the University of Chicago, the Massachusetts Institute of Technology and the University of Michigan.







No comments:
Post a Comment