From Wikipedia, the free encyclopedia
This article is an accessible, non-technical introduction to the subject. For the main encyclopedia article, see Angular momentum.
In physics, angular momentum is the rotational counterpart of linear momentum. A freely-rotating disk (like a Frisbee in flight or a tire rolling down a hill) has angular momentum.
It is a vector quantity, meaning it has both direction and magnitude. The magnitude L of an object's angular momentum is
In the SI system of units, angular momentum is measured in kg.m2.s−1. In contrast, linear momentum is measured in units of kg.m.s−1 so the two are not compatible and cannot be added.
The angular momentum of a symmetrical body, such as a spinning flywheel, is product of the body's moment of inertia and its angular velocity.
To calculate the angular momentum of a rotating object (a rigid body like a wheel or a system of objects like the solar system), a point called the origin is chosen. For convenience, this is usually the axis of rotation (of a rigid body) or the center of mass (of a system). The distance from the origin to each part of the object is multiplied by the transverse component of the linear momentum of that part. The sum of these vector quantities is the object's angular momentum.
Contents
Examples
The following two examples, which were also very important in the history of physics, are very typical as cases where angular momentum is involved: the fact that a spinning upright gyroscope will remain upright and Kepler's second law, which is a case of conservation of angular momentum.
Kepler's second law. As a planet orbits the Sun equal areas are swept out in equal intervals of time.
The properties of angular momentum
Comparing angular momentum and momentum brings the properties of angular momentum into focus.| Angular momentum | Momentum |
|---|---|
The angular momentum of an object is defined relative to a fixed point. (In the case of a gyroscope the center of mass
is used as the fixed point, in the case of Kepler's second law the much
heavier Sun is used as the fixed point.) The amount of angular momentum
of an object with respect to a fixed point S is proportional to the object's moment of inertia  , and to the angular velocity relative to the fixed point. Multiplying those three factors gives the angular momentum. In notation: , and to the angular velocity relative to the fixed point. Multiplying those three factors gives the angular momentum. In notation:
|
The amount of momentum of an object is proportional to the object's mass, and to the velocity. Multiplying those two factors gives the momentum. In notation:
|
| The direction of angular velocity is defined with the help of the right hand rule. The direction of angular momentum is the same as the direction of the angular velocity. | The direction of momentum is the direction of the velocity. |
| In the absence of a torque both the amount and the direction of the angular momentum will not change. | In the absence of a force both the amount and direction of momentum will not change. |
| When two objects with a particular angular moment relative to a common fixed point S interact they affect each other's angular momentum; in this interaction the total angular momentum is conserved. Example: Gravitational slingshot | When two objects interact (in a collision for example), they affect each other's momentum; in this interaction the total momentum is conserved. |
Newton's derivation of the area law
Newton's geometric derivation of the area law from the laws of motion: as an object orbits the Sun it sweeps out equal areas in equal intervals of time. [1] [2] The picture is adapted from Newton's original diagram, the underlying mathematics is the same.An object (not shown) is in orbit around the Sun (point S). The effect of the Sun's gravitational attraction is represented as a sequence of instantaneous impulses, always directed towards point S. In the limit of the time interval between the impulses approaching to zero the sequence of impulses approaches infinitely close to continuous gravitational attraction.
When the object is at point B it receives an impulse towards point S. Without that impulse the object would proceed to point c. The actual displacement BC follows from the rules for velocity composition; the displacement BC is the vector sum of the displacements BV and Bc. The triangles SBc and SBC have the same base and the same height, hence they have the same surface area.
At point C the object receives another impulse towards point S. Without that impulse the object would have proceeded to point d in an equal interval of time. The impulse towards point S makes the object proceed to point D.
The triangles SBc, SBC, SCd, SCD, SDe, SDE all have the same surface area.
In the limit of the time intervals going to infinitisimally small the sequence of lines connecting the points B, C, D, E, etc. approaches infinitely close to the actual continuous trajectory.
Note that this derivation proves a more general law than Kepler's law of areas. This derivation shows that the area law applies for any central force, not just for gravity.
The area law as a case of angular momentum conservation.
That angular momentum is proportional to area swept out can be understood as follows: in the case of triangle SBC the length SB is equal to r, the distance to the fixed point. The length BC correlates with the angular velocity relative to point S. Multiplying the angular velocity ω with r gives the length BC. Hence the area is proportional to r*r*ω, and angular momentum is proportional to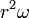
Angular momentum involves a squared form:
 . This is why in a geometrical representation angular momentum is proportional to an area rather than to a length.
. This is why in a geometrical representation angular momentum is proportional to an area rather than to a length.Importance in the Principia
In Newton's major work, the Philosophiae Naturalis Principia Mathematica the above derivation is presented in Book I, Proposition I, Theorem I. Having the area law derived from first principles made it possible for Newton to represent the passage of time geometrically, which greatly enhanced Newton's possibilities for computations and proofs.General concept of angular momentum
Main article: Angular momentum
The above description of angular momentum was restricted to a
specific class of cases: the cases where the fixed point lies in the
plane of rotation. This can be thought of as a planar version of angular
momentum, only two dimensions of space are considered.More generally the fixed point can be placed outside the plane of rotation. In this generalized concept of angular momentum all three of the spatial dimensions are considered.



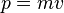

No comments:
Post a Comment